Why Choose Us?
Discover Our Unique Approaches to Health
01.
Evidence-Based Insights
We provide health information rooted in scientific research, ensuring that our advice is safe, effective, and relevant for all users.
02.
Inclusive Community Support
Join a supportive online community where individuals can share experiences, find motivation, and encourage each other’s wellness journeys.
03.
Up-to-Date Information
Our content is continually updated to reflect the latest health trends, research, and wellness strategies, keeping you informed and engaged.

Blog
Speech Therapy at Home: Is Remote Treatment Effective for Kids?
Introduction to Speech Therapy Speech therapy is a vital intervention for children experiencing communication difficulties. These challenges can arise from various conditions, including developmental delays, neurological disorders, or speech impediments. Traditionally, speech therapy has been conducted in person, with therapists working directly with children in clinical settings. However, the advent of technology has paved the...
Read MoreKids Speech Therapy Near Me: 3 Questions Every Parent Should Ask
Finding the right speech therapy for children can be a daunting task for parents. With numerous options available, it’s essential to ask the right questions to ensure that your child receives the best possible care. This article will explore three critical questions that every parent should consider when seeking speech therapy services for their child...
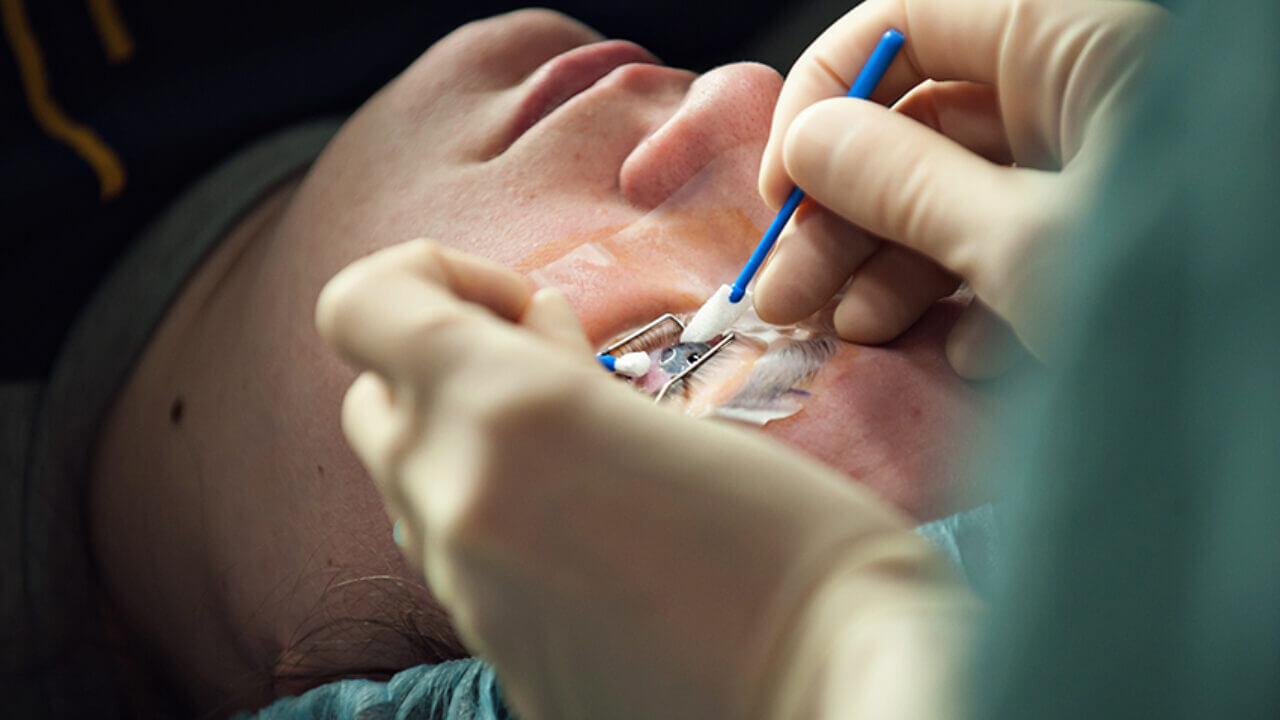
Read MoreHow Much Is LASIK in Australia? Realistic Budgets and Expectations
Understanding LASIK Surgery LASIK, or Laser-Assisted In Situ Keratomileusis, is a popular eye surgery designed to correct refractive vision issues such as myopia, hyperopia, and astigmatism. This procedure uses advanced laser technology to reshape the cornea, allowing light to focus correctly on the retina. For many Australians, LASIK represents a chance to achieve clearer vision...
Read MoreFinding the Best Lasik Eye Surgery Cost Sydney: What to Look For
Lasik eye surgery has become a popular option for those seeking to correct vision problems, offering a chance to live life without the hassle of glasses or contact lenses. However, one of the most significant considerations for prospective patients is the cost associated with the procedure. In Sydney, where the options for Lasik surgery are...
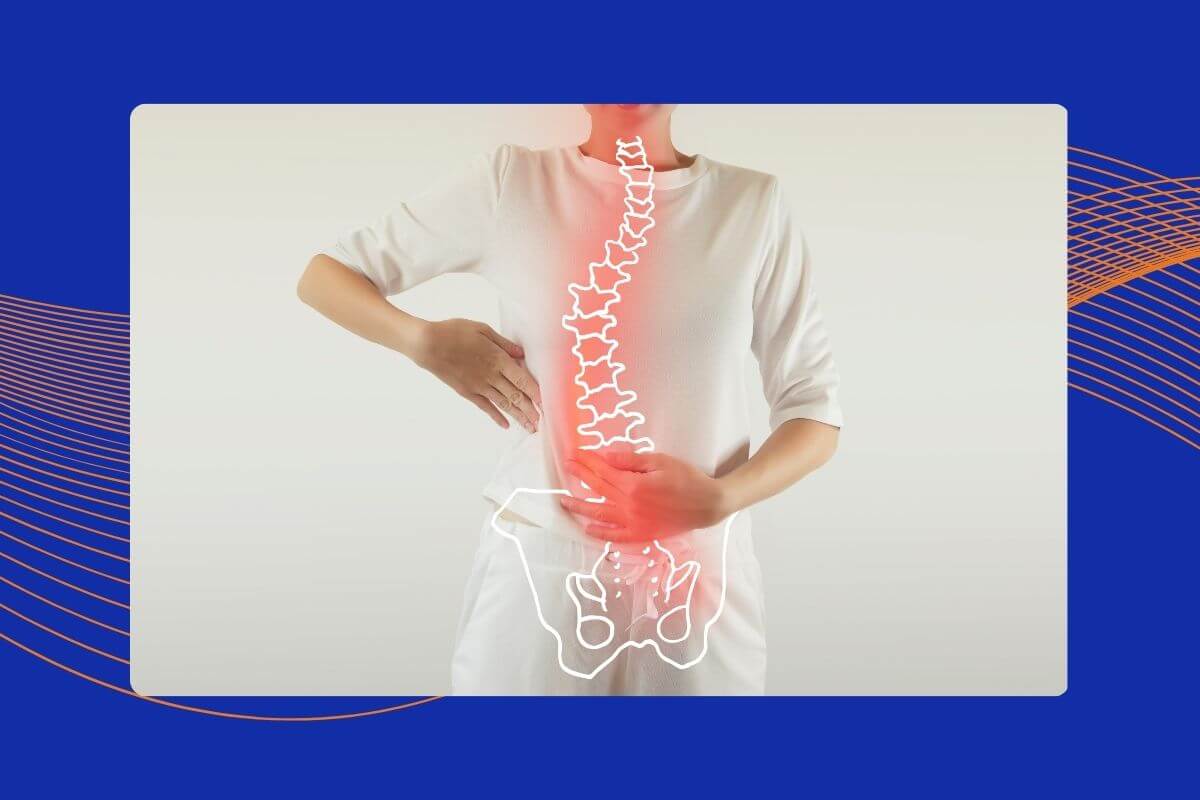
Read MoreScoliosis Management: Effective Exercises to Consider
Scoliosis, a medical condition characterised by an abnormal curvature of the spine, can be challenging to manage. While treatment options vary depending on the severity of the condition, exercises have been proven to be an effective method for improving the symptoms of scoliosis. From targeted stretches to strength-building exercises, we will delve into the specifics...
Read MoreSCL Health Landing
I always have to stress that this is not a ‘health landing’. This is a landing that we all need. The last thing we want to do is ruin our health, but we all need to make sure that we are doing all of our health-related actions with purpose and focus. Whether you’re just starting...
Read MoreWhat Should Be Done Following Health Violations From An Inspection
From the inspector’s perspective, it is important to be aware of the health violations that may have occurred during the inspection. If a home is not up to code, it can be very difficult to work with a home inspector who may not be familiar with the home’s code. Even if you are the home...
Read MoreDark Souls Boss Health Bar Template
If you have a dark soul in your life, you probably have a “boss” that you feel needs to be taken care of. This boss can be you, a person you work with, or your kids and family. The key is to know who that boss is and have a plan B. Use this template...
Read MoreWhat We Offer
Explore Our Health and Wellness Resources



What People Say
Testimonials from Our Readers
Healthy Times Online has completely transformed the way I approach my health and fitness. The articles are easy to understand and incredibly helpful!
I love how accessible the advice is! It’s made a big difference in my family’s eating habits and overall wellness. Highly recommend!

Why Choose Us?
Unmatched Reliability and Support
Discover how we differentiate ourselves in the health and wellness space by providing trustworthy, inclusive, and up-to-date information.